How to Determine if a Set Is Countable

If a subset of a set is uncountable then the set is uncountable. The following three conditions are equivalent.

1 11 3 Countable Sets Video Youtube

2 There is an injection f.

. 1 S is countable. In practise we will often just say countable when we really mean countably in nite when it is clear that the set involved is in nite. Let h1 minA.

How do you know if a set is countable. Note that R A T and A is countable. As in the first one and as in the second are quite different.

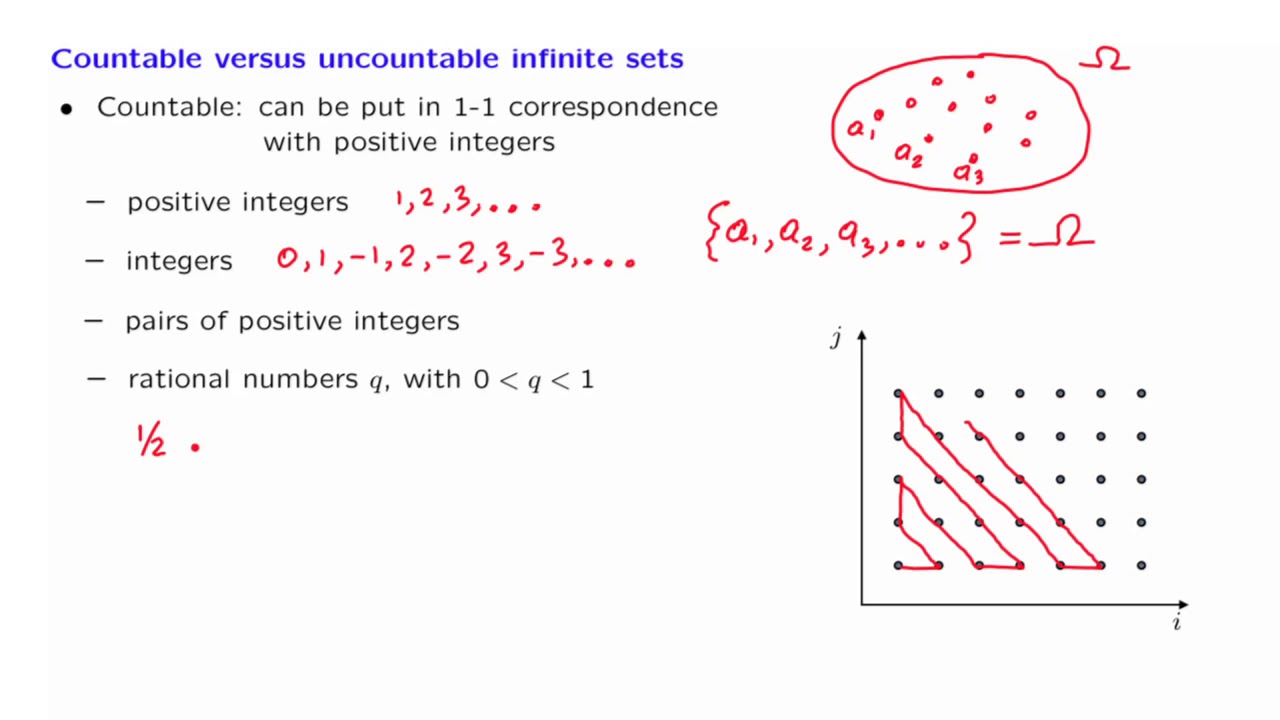
So a proof of countability amounts to providing a function that maps natural numbers to the set and then proving it is surjective. In mathematics a set is countable if it has the same cardinality the number of elements of the set as some subset of the set of natural numbers N 0 1 2 3. S N from S to the natural numbers N 0 1 2 3.

Using this concept we may summarize some of our above results as follows for a non-empty set. The real numbers between 0and scan be listed in order r 1r 2r 3. 1 S is countable.

The following three conditions are equivalent. It can be put in one-to-one correspondence with natural numbers mathbb N in which case the set is said to be countably infinite. Let S be a nonempty set.

3 There is a surjection g. 2 There is an injection f. Since A is infinite A is nonempty and so h is well-defined.

Having defined hn 1 we define hn minAh1hn1. It simply means that every element in S corresponds to a different element in N. We define h.

If T were countable then R would be the union of two countable sets. We will show that 1 implies 2 implies 3. Any subset of a countable set is countable.

3 There is a surjection g. First we prove a. Equivalently a set S is countable if there exists an injective function f.

A set is countably infinite if it is countable and infinite just like the positive integers. Since R is un-countable R is not the union of two countable sets. A useful criterion to determine a set is countable A set is countable if it is either countably in nite or nite.

Let S be a nonempty set. A set A is countably infinite if its cardinality is equal to the cardinality of the natural numbers N. The correspondence as you stated is n 2n.

N A as follows. A fA by setting fx fx for every x A. A countable set is either a finite set or a countably infinite set.

In words a set is countable if it has the same cardinality as some subset of the natural numbers. By a list we mean that you can find a first member a second one and so on and eventually assign to each member an integer of its own perhaps going on forever. If it is a finite set mid A mid infty.

A set is countable if it can be placed in surjective correspondence with the natural numbers. The cardinality of a subset is at least as large as the cardinality of the entire set. Then the real numbers between 0and sare also countable any subset of a countable set is countable 2.

Since fA is a subset of the countable set B it is countable and therefore so is A. The natural numbers are themselves countable- you can assign each integer to itself. The set of real numbers is uncountable.

Let A denote the set of algebraic numbers and let T denote the set of tran-scendental numbers. A useful criterion to determine a set is countable A set is countable if it is either countably in nite or nite. A set Ais said to be countably in nite if jAj jNj and simply countable if jAj jNj.

A set is countable if it can be put into a one-to-one correspondence with the natural numbers or some subset of the natural numbers Some classes only accept infinite sets as countable others include finite sets. S N from S to N. Set A is called countable if one of the following is true.

By definition a set S is countable if there exists an injective function f. In mathematics a set is countable if it has the same cardinality as some subset of the set of natural numbers N 0 1 2 3. It is enough to prove that there is a subset of R that is.

N 1 2 and Even 2 4 6 have the same cardinality because there is one to one correspondence from N onto Even. Without loss of generality we may assume that A is an infinite subset of N. A set is countable if it is finite or it can be placed in 1-1 correspondence with the positive integers.

In the latter case is said to be countably infinite. Suppose B is countable and there exists an injection f. Again since A is infinite the set.

Countable sets A set is said to be countable if and only if it is either finite or there is a bijection from to. The nonnegative integers are countable as shown by the bijection f n. Just as in the proof of Theorem 4 on the finite sets handout we can define a bijection f.

Second you need to read the question carefully. A set is uncountable if it is infinite and not countably infinite. If a set is not countable then is said to be uncountable.

The method is called the Cantor diagonalization argument and is a proof by contradiction. We will show that 1 implies 2 implies 3 implies 1. A set is said to be countable if you can make a list of its members.

Introduction To The Cardinality Of Sets And A Countability Proof Cardinality Math Videos Introduction

S01 8 Countable And Uncountable Sets Youtube

Elementary Set Theory On Countable Sets Mathematics Stack Exchange

An Easy Proof That Rational Numbers Are Countable